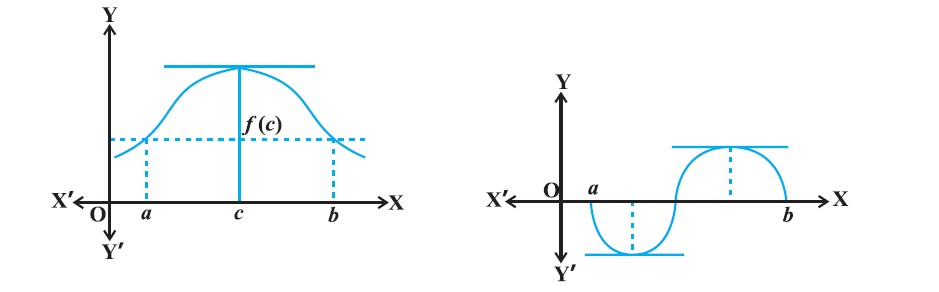
`\color{green} ✍️` Let `f : [a, b] → R` be a continuous function on `[a, b]` and differentiable on `(a, b)`.
`\color{green} ✍️` Then there exists some `c` in `(a, b)` such that
`color{red}{f'(c) =(f(b)-f(a))/(b-a)}`
`=>` Mean Value Theorem (MVT) is an extension of Rolle’s theorem.
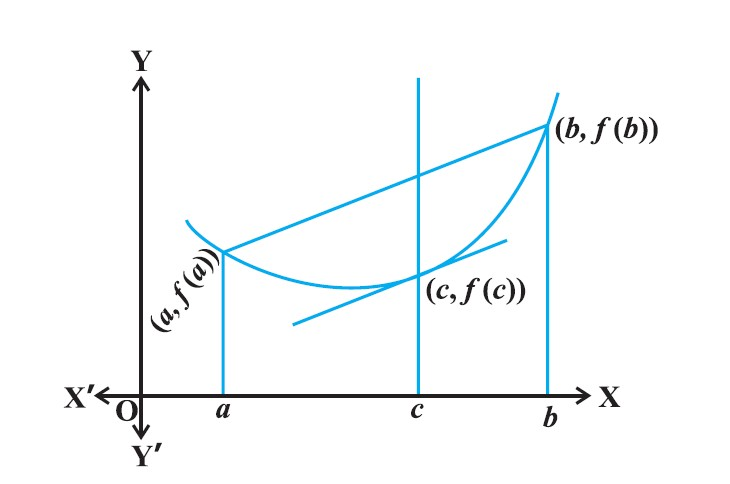
`=>` In fig `y = f(x)` is given, We've already interpreted `f ′(c)` as the slope of the tangent to the curve `y = f (x)` at `(c, f (c))`.
`\color{green} ✍️` From the Fig `color{blue}{((f(b)-f(a))/(b-a))}` is the slope of the secant drawn between `(a, f (a))` and `(b, f (b))`.
`=>` `color{blue}{"The MVT states that there is a point" \ \ c \ \ "in" (a, b) "such that the slope of the tangent at"}`
`color{blue}{ (c, f(c)) "is same as the slope of the secant between" (a, f (a)) "and" (b, f (b))}`.
`\color{green} ✍️` In other words, there is a point `c` in `(a, b)` such that the tangent at `(c, f (c))` is parallel to the secant between `(a, f (a))` and `(b, f (b))`.
`\color{green} ✍️` Let `f : [a, b] → R` be a continuous function on `[a, b]` and differentiable on `(a, b)`.
`\color{green} ✍️` Then there exists some `c` in `(a, b)` such that
`color{red}{f'(c) =(f(b)-f(a))/(b-a)}`
`=>` Mean Value Theorem (MVT) is an extension of Rolle’s theorem.
`=>` In fig `y = f(x)` is given, We've already interpreted `f ′(c)` as the slope of the tangent to the curve `y = f (x)` at `(c, f (c))`.
`\color{green} ✍️` From the Fig `color{blue}{((f(b)-f(a))/(b-a))}` is the slope of the secant drawn between `(a, f (a))` and `(b, f (b))`.
`=>` `color{blue}{"The MVT states that there is a point" \ \ c \ \ "in" (a, b) "such that the slope of the tangent at"}`
`color{blue}{ (c, f(c)) "is same as the slope of the secant between" (a, f (a)) "and" (b, f (b))}`.
`\color{green} ✍️` In other words, there is a point `c` in `(a, b)` such that the tangent at `(c, f (c))` is parallel to the secant between `(a, f (a))` and `(b, f (b))`.